Is -2 Pi A Rational Number​?

‍

Mathematics applies an exclusive system to organize numbers by their noticeable traits. Science and math draw considerable value from two number categories: rational and irrational numbers. 

The question sometimes emerges about whether the number -2π belongs to the rational number category. 

Before determining whether -2π falls into a rational or irrational category, we must review their essential characteristics.

‍

What is a Rational Number?

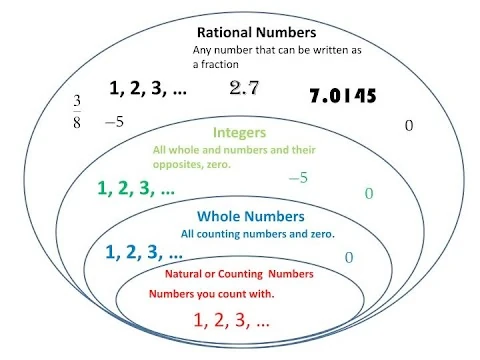
Rational numbers take the form of fractions connecting two integers. When written as the number meets rational requirements.

‍

 r = a/b

‍

Where a and b are fractions and b ≄ 0, examples of rational numbers are:

‍

• The rational number 3 finds expression through the fraction combination of its two integer parts.

‍

• Fractions like 5/7

‍

• The number -4 takes this particular shape when written as -4/1.

‍

• You can find terminal or periodic decimal numbers in 0.75 and 0.333.

‍

No matter its position on the number line, rational numbers share the quality of appearing as a fraction of two decimals.

What is an irrational number?

‍

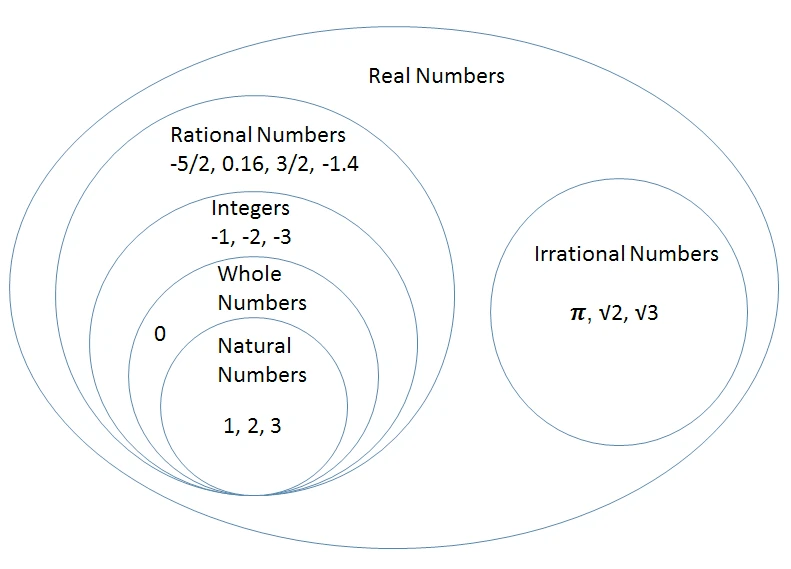
An irrational number is opposite to a rational number because it is impossible to show it as a simple division between two numbers. The decimal representation of irrational numbers does not end or repeat. 

‍

Numerical expressions make up typical irrational numbers that are:

‍

• ⟌2
• The number e defines natural logarithms' fundamental value
• The most famous irrational number, 𝜋

‍

In mathematics, “𝜋” stands as a substantial number and defines itself as the constant that links a circle's diameter to its circumference. Since 𝜋 is defined as a ratio, it needs to be represented using integers, but it cannot function as fractional parts of integers. The decimal places of pi extend infinitely without following any repeating numbers to qualify as an irrational number.

‍

Acknowledging -2𝜋

We will use our understanding of rational and irrational numbers to study -2π.

‍

When multiplying -2 with 𝜋, the result is -2𝜋. Since we understand “𝜋 is irrational,” our next task is to identify how negative two changes its class. 

‍

These are the points we need to assess.

1. Multiplication & Irrationality: 

A product of rational and irrational numbers will always be an irrational number. Throughout this example, -2 functions as a rational number that yields -2/1.

2. Product of -2 and 𝜋:

The product of -2 and 𝜋 remains irrational since the result cannot be expressed as a fraction made from two whole numbers. The product stays irrational because 𝜋 keeps its irrational characteristics.

Why -2𝜋 is Irrational

We will prove -2π cannot be rational through this analysis.

‍

• The number -2𝜋 equals a rational number; there would exist integers a and b≄0, such as;

-2𝜋 = a/b

‍

• After rearranging this equation, we get

𝜋 = -a/2b

‍

• The result shows pi must be rational, which conflicts with standard knowledge that pi is irrational.

‍

Our conclusion stands that -2π is irrational because the opposite is true. By extension, -2π is irrational.

‍

Envisioning -2𝜋 

People who rely on common sense can view -2π as the opposite of the circle's double circumference divided by its diameter. Since pi works as an irrational value without achievable fraction representation, neither negative two pi nor other integer multiples would create fractions.

Frequently Asked Questions 

Why is Pi(𝜋) not a rational number?

The quantitative representation of Pi does not have a firm pattern, so it cannot be written as a fraction of whole numbers.

Is it difficult to understand 𝜋?

The complete understanding of 𝜋 proves hard because this irrational number shows an endless string of decimals that never loops back.

Is 0.3 an irrational number?

The number 0.3 fits into the category of rational numbers because it takes the form of a fraction division. 

‍

Conclusion

We would finalize that -2𝜋 is not a rational number. Since -2 times PI equals an irrational value PI, this result stays irrational. Studying irrational numbers shows us why -2π cannot be converted into simple fractions and rational values. Our knowledge of mathematical relationships is enhanced when we know how sensible and irrational numbers differ.